Leetcode 337. 打家劫舍 III(DAY 88) ---- Leetcode Hot 100
本文共 1034 字,大约阅读时间需要 3 分钟。
原题题目
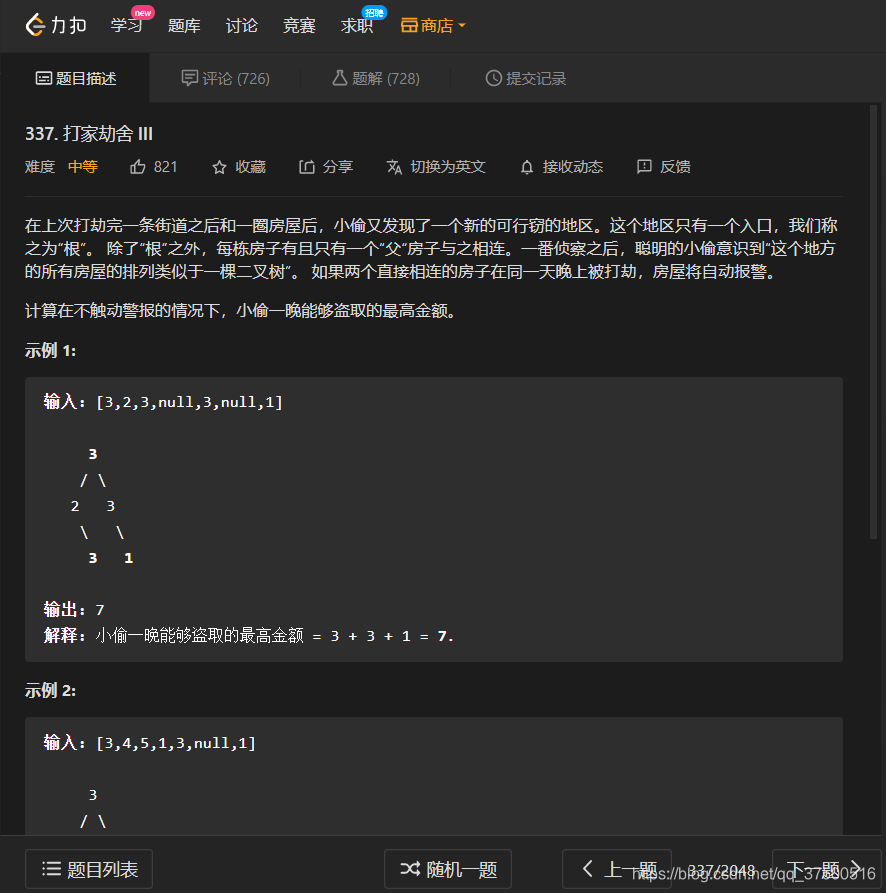
代码实现(首刷自解 之前没做明白 说明3个月的提升aaa)
/** * Definition for a binary tree node. * struct TreeNode { * int val; * TreeNode *left; * TreeNode *right; * TreeNode() : val(0), left(nullptr), right(nullptr) {} * TreeNode(int x) : val(x), left(nullptr), right(nullptr) {} * TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {} * }; */class Solution { public: unordered_map m; int rob(TreeNode* root) { if(!root) return 0; if(!root) return 0; int l = rob(root->left); int r = rob(root->right); int ll = 0,lr = 0,rl = 0,rr = 0; if(root->left) { ll = m[root->left->left]; lr = m[root->left->right]; } if(root->right) { rl = m[root->right->left]; rr = m[root->right->right]; } if(l + r >= ll + lr + rl + rr + root->val) m[root] = l + r; else m[root] = ll + lr + rl + rr + root->val; return m[root]; }}; 转载地址:http://fcni.baihongyu.com/
你可能感兴趣的文章
Mysql学习总结(65)——项目实战中常用SQL实践总结
查看>>
Mysql学习总结(66)——设置MYSQL数据库编码为UTF-8
查看>>
Mysql学习总结(67)——MYSQL慢查询日志
查看>>
Mysql学习总结(68)——MYSQL统计每天、每周、每月、每年数据 SQL 总结
查看>>
Mysql学习总结(69)——Mysql EXPLAIN 命令使用总结
查看>>
Mysql学习总结(6)——MySql之ALTER命令用法详细解读
查看>>
Mysql学习总结(70)——MySQL 优化实施方案
查看>>
Mysql学习总结(71)——MySQL 重复记录查询与删除总结
查看>>
Mysql学习总结(71)——数据库介绍(MySQL安装 体系结构、基本管理)再回顾
查看>>
Mysql学习总结(72)——MySQL 开发者开发,设计规范再总结
查看>>
Mysql学习总结(73)——MySQL 查询A表存在B表不存在的数据SQL总结
查看>>
Mysql学习总结(74)——慢SQL!压垮团队的最后一根稻草!
查看>>
Mysql学习总结(75)——并发量大、数据量大的互联网业务数据库设计军规
查看>>
Mysql学习总结(76)——MySQL执行计划(explain)结果含义总结
查看>>
Mysql学习总结(77)——温故Mysql数据库开发核心原则与规范
查看>>
Mysql学习总结(78)——MySQL各版本差异整理
查看>>
Mysql学习总结(79)——MySQL常用函数总结
查看>>
Mysql学习总结(7)——MySql索引原理与使用大全
查看>>
Mysql学习总结(80)——统计数据库的总记录数和库中各个表的数据量
查看>>
Mysql学习总结(81)——为什么MySQL不推荐使用uuid或者雪花id作为主键?
查看>>